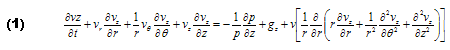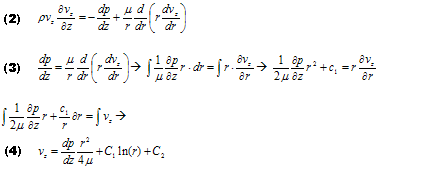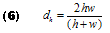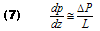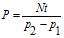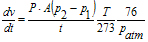PDMS Viscometers: Using Microfluidic Technologies for Everyday Applications

How can fluid velocity determine viscosity?

A fluid's viscosity can be determined by its velocity through a channel using the Hagen Poiseuille equation.

Derivation of Hagen- Poiseuille Equation

The Hagen-Poiseuille Equation is an exact solution of the Navier-Stokes equations for incompressible flow in a straight channel. Since the Navier-Stokes equations are second-order nonlinear partial differential equations any analytic solution can be quite useful. The complete derivation is arduous and can be found in a number of references. In order to correctly derive the Hagen-Poiseuille equation several assumptions must be met:

Fully developed flow; the point of measurement is assumed to be far from any channel entrance, sidewall etc… so that flow is purely axial
Incompressible fluid
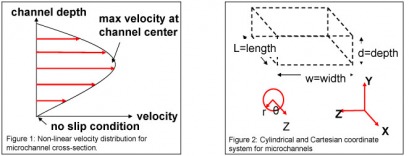
No-slip condition; flow at the channel walls is zero (see Figure 2)
No swirl and no radial velocity components
Gravitational effects ignored
Steady flow
Newtonian fluid (constant viscosity)
Note: derivation is performed in cylindrical coordinates (see Figure 1) 

The generalized Navier-Stokes equation (axially and radial momentum components see figure 2):

Assuming steady flow, no radial momentum,  no horizontal flow,  and that flow is fully developed  (1) reduces to:      

The boundary conditions of; no slip at the channel wall and a finite velocity at the centerline can be substituted into both constants. For the no-slip condition distance measured from the centerline (r) equals the channel wall radius (R). To find the finite velocity at the channel’s center (r) must equal zero. Inserting these two boundary conditions yields the Hagen Poiseuille equation:

This equation will yield various velocity values along a circular cross-section of a straight pipe, but our PDMS channels are rectangular and only the maximum velocity can be visually measured. Several modifications to {7} need to be made in order to establish an accurate relationship between fluid velocity and viscosity. 

 We will assume that the fluid velocity is measured at the channel centerline so that (r)=0. To account for the square cross-section R is replaced with hydraulic diameter where;

Since the pressure drop as a function of channel length and not a function of width (r) the channel pressure drop can be approximated by:

Lastly, an additional correction must be made to account for the square channel geometry so that the final Hagen-Poiseuille equation for a microfluidic channel is:

How is a vacuum developed inside the PDMS channels?

Permeability of PDMS

PDMS is highly permeable to gases and solvents which gives it some useful properties for designing microfluidic devices. Absorption driven devices have been recently studied since they allow for an “on chip” pumping apparatus, deliver uniform flow rates for a suitable time scale, are cheap and easy to manufacture, and compliment the disposable nature of microfluidic device structures.

High permeability is desirable to drive a significant volume of air from the PDMS structure with modest vacuum pressures (10-50 kPa) and short (15-60 min) times. However, this high permeability leads to significant diffusion fluid losses for certain types of fluids, particularly organic solvents. This high diffusion process results in slower fluid velocities since the fluid has axial and horizontal direction vectors. Solvents such as toluene cause the inner channel walls to swell, thus reducing hydraulic diameter which increases capillary pressure or can pinch off flow altogether. The PDMS viscometer can not accurately measure organic solvent viscosities for these reasons.

The large surface area of the PDMS viscometer coupled with the small channel volumes results in a significant pressure differential developed between the inner fluid /air interface and atmosphere. Permeability, pressure differentials, time and gas flux can be related by:

where:

P = permeability coefficient in Barrers
P2 = feed pressure
P1 = permeate pressure
N = steady state gas flux

For two dimensional steady state gas flux the flow rate can be related to area and pressure differential by:

where:
Velocity = cubic centimeters per second
A = area in square centimeters
T = temperature in Kelvin

This equation can be used to estimate the fluid velocity inside the viscometer, while neglecting capillary force contributions to fluid velocity.